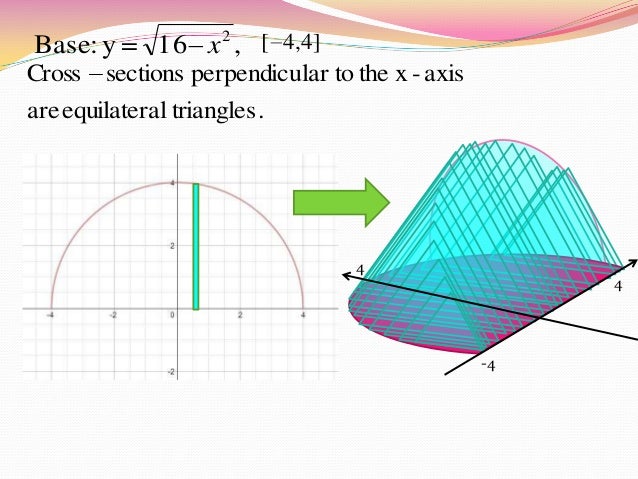
11.4 Perpendicular Cross Sectionsap Calculus
- 11.4 Perpendicular Cross Sections Ap Calculus Formulas
- 11.4 Perpendicular Cross Sectionsap Calculus Solver
- 11.4 Perpendicular Cross Sectionsap Calculus Equation
- 11.4 Perpendicular Cross Sectionsap Calculus Formulas
- 11.4 Perpendicular Cross Sections Ap Calculus Multiple Choice
For exercises 1-4, the vectors (vecs{u}) and (vecs{v}) are given.
a. Find the cross product (vecs{u}timesvecs{v}) of the vectors (vecs{u}) and (vecs{v}). Express the answer in component form.
b. Sketch the vectors (vecs{u}, , vecs{v}), and (vecs{u}timesvecs{v}).
1) (quad vecs{u}=⟨2,0,0⟩, quad vecs{v}=⟨2,2,0⟩)
Calculus 3 Lecture 11.4: The Cross Product: Finding the Cross Product of two vectors with Determinants, Using the Cross Product to find Mutually Orthogonal. If the cross sections are perpendicular to the y‐axis, then their areas will be functions of y, denoted by A(y). In this case, the volume ( V ) of the solid on a, b is Example 1: Find the volume of the solid whose base is the region inside the circle x 2 + y 2 = 9 if cross sections taken perpendicular to the y ‐axis are squares. From calculus, we know the volume of an irregular solid can be determined by evaluating the following integral: Where A(x) is an equation for the cross-sectional area of the solid at any point x. We know our bounds for the integral are x=1 and x=4, as given in the problem, so now all we need is to find the expression A(x) for the area of our solid. Finding a Point of IntersectionFind the point of intersection of the plane 3 x − y + 4 z = 7 and the line through ( 5, 4, − 3 ) that is perpendicular to this plane. Point D is the other side of the line AC, the same distance from (10,6) as B (11,4) is (10,6) is the midpoint between B and D, the average of their coordinates. 10 to 11 = 1 increase, so decrease 10 one to get 9 as the x coordinate of D. 6 to 4 = 2 decrease, so increase 6 by 2 to get 8.
(b.)
2) (quad vecs{u}=⟨3,2,−1⟩, quad vecs{v}=⟨1,1,0⟩)
3) (quad vecs{u}=2hat{imath}+3hat{jmath}, quad vecs{v}=hat{jmath}+2hat{k})
(b.)
4) (quad vecs{u}=2hat{jmath}+3hat{k}, quad vecs{v}=3hat{imath}+hat{k})
5) Simplify ((hat{imath}×hat{imath}−2hat{imath}×hat{jmath}−4hat{imath}×hat{k}+3hat{jmath}×hat{k})×hat{imath}.)
- Answer:
- (−2hat{jmath}−4hat{k})
6) Simplify (hat{jmath}×(hat{k}×hat{jmath}+2hat{jmath}×hat{imath}−3hat{jmath}×hat{jmath}+5hat{imath}×hat{k}).)
In exercises 7-10, vectors (vecs{u}) and (vecs{v}) are given. Find unit vector (vecs{w}) in the direction of the cross product vector (vecs{u}×vecs{v}.) Express your answer using standard unit vectors.
7) (quad vecs{u}=⟨3,−1,2⟩, quad vecs{v}=⟨−2,0,1⟩)
- Answer:
- (vecs{w}=−frac{sqrt{6}}{18}hat{imath}−frac{7sqrt{6}}{18}hat{jmath}−frac{sqrt{6}}{9}hat{k})
8) (quad vecs{u}=⟨2,6,1⟩, quad vecs{v}=⟨3,0,1⟩)
9) (quad vecs{u}=vecd{AB}, quad vecs{v}=vecd{AC},) where (A(1,0,1), B(1,−1,3)), and (C(0,0,5))
- Answer:
- (vecs{w}=−frac{4sqrt{21}}{21}hat{imath}−frac{2sqrt{21}}{21}hat{jmath}−frac{sqrt{21}}{21}hat{k})
10) (quad vecs{u}=vecd{OP}, quad vecs{v}=vecd{PQ},) where (P(−1,1,0)) and (Q(0,2,1))
11) Determine the real number (α) such that (vecs{u}timesvecs{v}) and (hat{imath}) are orthogonal, where (vecs{u}=3hat{imath}+hat{jmath}−5hat{k}) and (vecs{v}=4hat{imath}−2hat{jmath}+αhat{k}.)
- Answer:
- (α=10)
12) Show that (vecs{u}timesvecs{v}) and ( 2hat{imath}−14hat{jmath}+2hat{k}) cannot be orthogonal for any α real number, where (vecs{u}=hat{imath}+7hat{jmath}−hat{k}) and (vecs{v}=αhat{imath}+5hat{jmath}+hat{k}).
13) Show that (vecs{u}timesvecs{v}) is orthogonal to (vecs{u}+vecs{v}) and (vecs{u}−vecs{v}), where (vecs{u}) and (vecs{v}) are nonzero vectors.
14) Show that (vecs{v}timesvecs{u}) is orthogonal to ( (vecs{u}⋅vecs{v})(vecs{u}+vecs{v})+vecs{u}), where (vecs{u}) and (vecs{v}) are nonzero vectors.
15) Calculate the determinant ( begin{bmatrix}hat{imath}&hat{jmath}&hat{k}1&−1&72&0&3end{bmatrix}).
- Answer:
- ( −3hat{imath}+11hat{jmath}+2hat{k})
16) Calculate the determinant ( begin{bmatrix}hat{imath}&hat{jmath}&hat{k}0&3&−41&6&−1end{bmatrix}).
For exercises 17-18, the vectors (vecs{u}) and (vecs{v}) are given. Use determinant notation to find vector (vecs{w}) orthogonal to vectors (vecs{u}) and (vecs{v}).
17) (quad vecs{u}=⟨−1, 0, e^t⟩, quad vecs{v}=⟨1, e^{−t}, 0⟩,) where (t) is a real number
- Answer:
- (vecs{w}=⟨−1, e^t, −e^{−t}⟩)
Fly_piganne 28 online, free games to play. 18) (quad vecs{u}=⟨1, 0, x⟩, quad vecs{v}=⟨frac{2}{x},1, 0⟩,) where (x) is a nonzero real number
19) Find vector ( (vecs{a}−2vecs{b})×vecs{c},) where ( vecs{a}=begin{bmatrix}hat{imath}&hat{jmath}&hat{k}2&−1&50&1&8end{bmatrix}, vecs{b}=begin{bmatrix}hat{imath}&hat{jmath}&hat{k}0&1&12&−1&−2end{bmatrix},) and (vecs{c}=hat{imath}+hat{jmath}+hat{k}.)
- Answer:
- ( −26hat{imath}+17hat{jmath}+9hat{k})
20) Find vector ( vecs{c}×(vecs{a}+3vecs{b}),) where ( vecs{a}=begin{bmatrix}hat{imath}&hat{jmath}&hat{k}5&0&90&1&0end{bmatrix}, vecs{b}=begin{bmatrix}hat{imath}&hat{jmath}&hat{k}0&−1&17&1&−1end{bmatrix},) and (vecs{c}=hat{imath}−hat{k}.)
21) [T] Use the cross product (vecs{u}timesvecs{v}) to find the acute angle between vectors (vecs{u}) and (vecs{v}), where (vecs{u}=hat{imath}+2hat{jmath}) and (vecs{v}=hat{imath}+hat{k}.) Express the answer in degrees rounded to the nearest integer.
- Answer:
- ( 72°)
22) [T] Use the cross product (vecs{u}timesvecs{v}) to find the obtuse angle between vectors (vecs{u}) and (vecs{v}), where (vecs{u}=−hat{imath}+3hat{jmath}+hat{k}) and (vecs{v}=hat{imath}−2hat{jmath}.) Express the answer in degrees rounded to the nearest integer.
23) Use the sine and cosine of the angle between two nonzero vectors (vecs u) and (vecs v) to prove Lagrange’s identity: (|vecs{u}timesvecs{v}|^2=|vecs{u}|^2|vecs{v}|^2−(vecs{u}⋅vecs{v})^2).
24) Verify Lagrange’s identity (|vecs{u}timesvecs{v}|^2=|vecs{u}|^2|vecs{v}|^2−(vecs{u}⋅vecs{v})^2) for vectors (vecs{u}=−hat{imath}+hat{jmath}−2hat{k}) and (vecs{v}=2hat{imath}−hat{jmath}.)
25) Nonzero vectors (vecs{u}) and (vecs{v}) are called collinear if there exists a nonzero scalar (α) such that ( vecs{v}=αvecs{u}). Show that (vecs{u}) and (vecs{v}) are collinear if and only if ( vecs{u}timesvecs{v}=0.)
26) Nonzero vectors (vecs{u}) and (vecs{v}) are called collinear if there exists a nonzero scalar (α) such that ( vecs{v}=αvecs{u}). Show that vectors ( vecd{AB}) and (vecd{AC}) are collinear, where (A(4,1,0), , B(6,5,−2),) and (C(5,3,−1).)
27) Find the area of the parallelogram with adjacent sides (vecs{u}=⟨3,2,0⟩) and (vecs{v}=⟨0,2,1⟩).
- Answer:
- (7)
28) Find the area of the parallelogram with adjacent sides (vecs{u}=hat{imath}+hat{jmath}) and (vecs{v}=hat{imath}+hat{k}.)
29) Consider points (A(3,−1,2), B(2,1,5),) and (C(1,−2,−2).)
a. Find the area of parallelogram ABCD with adjacent sides (vecd{AB}) and ( vecd{AC}).
b. Find the area of triangle ABC.
c. Find the distance from point A to line BC.
- Answer:
- a. (5sqrt{6};) b. (frac{5sqrt{6}}{2};) c. (frac{5sqrt{6}}{sqrt{59}} =frac{5sqrt{354}}{59} )
30) Consider points (A(2,−3,4), B(0,1,2),) and (C(−1,2,0).)
a. Find the area of parallelogram ABCD with adjacent sides ( vecd{AB}) and ( vecd{AC}).
b. Find the area of triangle ABC.
c. Find the distance from point B to line AC.
In exercises 31-32, vectors (vecs{u}, , vecs{v}), and (vecs{w}) are given.
a. Find the triple scalar product (vecs{u}⋅(vecs{v}×vecs{w}).)
b. Find the volume of the parallelepiped with the adjacent edges (vecs{u},vecs{v}), and (vecs{w}).
31) (quad vecs{u}=hat{imath}+hat{jmath}, quad vecs{v}=hat{jmath}+hat{k},) and (quad vecs{w}=hat{imath}+hat{k})
- Answer:
- ( a. 2; quad b. 2) units3
32) (quad vecs{u}=⟨−3,5,−1⟩, quad vecs{v}=⟨0,2,−2⟩,) and (quad vecs{w}=⟨3,1,1⟩)
33) Calculate the triple scalar products (vecs{v}⋅(vecs{u}×vecs{w})) and (vecs{w}⋅(vecs{u}×vecs{v}),) where (vecs{u}=⟨1,1,1⟩, vecs{v}=⟨7,6,9⟩,) and (vecs{w}=⟨4,2,7⟩.)
- Answer:
- (vecs{v}⋅(vecs{u}×vecs{w})=−1, quad vecs{w}⋅(vecs{u}×vecs{v})=1)
34) Calculate the triple scalar products (vecs{w}⋅(vecs{v}×vecs{u})) and (vecs{u}⋅(vecs{w}×vecs{v}),) where (vecs{u}=⟨4,2,−1⟩, vecs{v}=⟨2,5,−3⟩,) and (vecs{w}=⟨9,5,−10⟩.)
35) Find vectors (vecs{a}, vecs{b}), and (vecs{c}) with a triple scalar product given by the determinant ( begin{bmatrix}1&2&30&2&58&9&2end{bmatrix}). Determine their triple scalar product.
- Answer:
- (vecs{a}=⟨1,2,3⟩, quad vecs{b}=⟨0,2,5⟩, quad vecs{c}=⟨8,9,2⟩; quad vecs{a}⋅(vecs{b}×vecs{c})=−9)
36) The triple scalar product of vectors (vecs{a},vecs{b}), and (vecs{c}) is given by the determinant ( begin{bmatrix}0&−2&10&1&41&−3&7end{bmatrix}). Find vector (vecs{a}−vecs{b}+vecs{c}.)
37) Consider the parallelepiped with edges ( OA,OB,) and ( OC), where ( A(2,1,0),B(1,2,0),) and ( C(0,1,α).)
a. Find the real number ( α>0) such that the volume of the parallelepiped is ( 3) units3.
b. For ( α=1,) find the height (h) from vertex (C) of the parallelepiped. Sketch the parallelepiped.
- Answer:
- ( a. , α=1; quad b. , h=1) unit,
38) Consider points ( A(α,0,0),B(0,β,0),) and ( C(0,0,γ)), with ( α, β), and ( γ) positive real numbers.
a. Determine the volume of the parallelepiped with adjacent sides ( vecd{OA}, vecd{OB},) and ( vecd{OC}).
b. Find the volume of the tetrahedron with vertices ( O,A,B,) and ( C). (Hint: The volume of the tetrahedron is ( 1/6) of the volume of the parallelepiped.)
c. Find the distance from the origin to the plane determined by ( A,B,) and ( C). Sketch the parallelepiped and tetrahedron.
11.4 Perpendicular Cross Sections Ap Calculus Formulas
39) Let ( u,v,) and ( w) be three-dimensional vectors and (c)be a real number. Prove the following properties of the cross product.
a. (vecs u×vecs u=vecs 0)
b. (vecs u×(vecs v+vecs w)=(vecs u×vecs v)+(vecs u×vecs w))
c. ( c(vecs u×vecs v)=(cvecs u)×vecs v=vecs u×(cvecs v))
d. ( vecs u⋅(vecs u×vecs v)=vecs 0)
40) Show that vectors (vecs u=⟨1,0,−8⟩,vecs v=⟨0,1,6⟩), and (vecs w=⟨−1,9,3⟩) satisfy the following properties of the cross product.
a. (vecs u×vecs u=vecs 0)
b. (vecs u×(vecs v+vecs w)=(vecs u×vecs v)+(vecs u×vecs w))
c. ( c(vecs u×vecs v)=(cvecs u)×vecs v=vecs u×(cvecs v))
d. (vecs u⋅(vecs u×vecs v)=vecs 0)
Puerto Rico is divided into 78 'municipios' (municipalities) which many states would refer to as counties.Those 78 municipalities are broken down into 'barrios'.These barrios are official, legal, geographical boundaries unlike in the states where a barrio may refer to a neighborhood such as Fairhill in Philadelphia or East Harlem in Manhattan. The purpose of Puerto Rico Genealogy is to provide information to beginners on how to find ancestors from Puerto Rico. I may include other parts of the world as Puerto Rico is a fusion of different cultures. I will also provide information about the history of Puerto Rico. I also want to share information related to Genetics. Puerto Rico Genealogy Puerto Rico Genealogy covers information about an island located in the Caribbean, that is just east of the island of Hispaniola and west of the Virgin Islands. Initially, the island was populated by indigenous people when Europeans arrived in 1493. Guide to Puerto Rico ancestry, family history and genealogy: birth records, marriage records, death records, census records, parish registers, and military records. Country Information edit edit source Puerto Rico is a United States Territory in the Caribbean whose nearest neighbors are the Dominican Republic, British Virgin Islands,. If you have a curiosity about Puerto Rico, its history, and/or your family history, or perhaps you have hit a brick wall, have no time to research, or simply need help finding an elusive ancestor simply ask us for help. We invite you to become a part of our growing community of researchers. (View samples of documents in our holdings). Pueblos of puerto ricopuerto rican genealogy.
41) Nonzero vectors (vecs u,vecs v), and (vecs w) are said to be linearly dependent if one of the vectors is a linear combination of the other two. For instance, there exist two nonzero real numbers ( α) and ( β) such that (vecs w=αvecs u+βvecs v). Otherwise, the vectors are called linearly independent. Show that (vecs u,vecs v), and (vecs w) could be placed on the same plane if and only if they are linear dependent.
42) Consider vectors (vecs u=⟨1,4,−7⟩,vecs v=⟨2,−1,4⟩,vecs w=⟨0,−9,18⟩), and (vecs p=⟨0,−9,17⟩.)
a. Show that (vecs u,vecs v), and (vecs w) can be placed on the same plane by using their triple scalar product
b. Show that (vecs u,vecs v), and (vecs w) can be placed on the same plane by using the definition that there exist two nonzero real numbers ( α) and ( β) such that ( w=αu+βv.)
c. Show that (vecs u,vecs v), and (vecs p) are linearly independent—that is, none of the vectors is a linear combination of the other two.
43) Consider points ( A(0,0,2), B(1,0,2), C(1,1,2),) and ( D(0,1,2).) Are vectors ( vecd{AB}, vecd{AC},) and ( vecd{AD}) linearly dependent (that is, one of the vectors is a linear combination of the other two)?
- Answer:
- Yes, ( vecd{AD}=αvecd{AB}+βvecd{AC},) where ( α=−1) and ( β=1.)
44) Show that vectors ( hat{imath}+hat{jmath}, hat{imath}−hat{jmath},) and ( hat{imath}+hat{jmath}+hat{k}) are linearly independent—that is, there exist two nonzero real numbers (α) and (β) such that (hat{imath}+hat{jmath}+hat{k}=α(hat{imath}+hat{jmath})+β(hat{imath}−hat{jmath}).)
45) Let (vecs u=⟨u_1,u_2⟩) and (vecs v=⟨v_1,v_2⟩) be two-dimensional vectors. The cross product of vectors (vecs u) and (vecs v) is not defined. However, if the vectors are regarded as the three-dimensional vectors ( tilde{vecs u}=⟨u_1,u_2,0⟩) and ( tilde{vecs v}=⟨v_1,v_2,0⟩), respectively, then, in this case, we can define the cross product of ( tilde{vecs u}) and ( tilde{vecs v}). In particular, in determinant notation, the cross product of ( tilde{vecs u}) and ( tilde{vecs v}) is given by
( tilde{vecs u}×tilde{vecs v}=begin{bmatrix}hatimath&hatjmath&hat{k}u_1&u_2&0v_1&v_2&0end{bmatrix}).
Use this result to compute ( (hatimathcos θ+hatjmathsin θ)×(hatimathsin θ−hatjmathcos θ),) where ( θ) is a real number.
- Answer:
- ( −hat{k})
46) Consider points ( P(2,1), Q(4,2),) and ( R(1,2).)
a. Find the area of triangle ( PQR).
b. Determine the distance from point ( R) to the line passing through ( P) and ( Q).
47) Determine a vector of magnitude ( 10) perpendicular to the plane passing through the x-axis and point ( P(1,2,4).)
- Answer:
- ( ⟨0,±4sqrt{5},2sqrt{5}⟩)
48) Determine a unit vector perpendicular to the plane passing through the z-axis and point ( A(3,1,−2).)
49) Consider (vecs u) and (vecs v) two three-dimensional vectors. If the magnitude of the cross product vector (vecs u×vecs v) is ( k) times larger than the magnitude of vector (vecs u), show that the magnitude of (vecs v) is greater than or equal to ( k), where ( k) is a natural number.
50) [T] Assume that the magnitudes of two nonzero vectors (vecs u) and (vecs v) are known. The function ( f(θ)=‖vecs u‖‖vecs v‖sin θ) defines the magnitude of the cross product vector (vecs u×vecs v,) where ( θ∈[0,π]) is the angle between (vecs u) and (vecs v).
a. Graph the function ( f).
b. Find the absolute minimum and maximum of function ( f). Interpret the results.
c. If ( ‖vecs u‖=5) and ( ‖vecs v‖=2), find the angle between (vecs u) and (vecs v) if the magnitude of their cross product vector is equal to ( 9).
51) Find all vectors (vecs w=⟨w_1,w_2,w_3⟩) that satisfy the equation ( ⟨1,1,1⟩×vecs w=⟨−1,−1,2⟩.) Hint: You should be able to write all components of (vecs w) in terms of one of the constants (w_1,w_2,) or (w_3).
- Answer:
- Writing all components in terms of the constant (w_3), one way to represent these vectors is: (vecs w=⟨w_3−1,w_3+1,w_3⟩,) where ( w_3) is any real number.
Note that we could use any parameter we wish here. We could set (w_3 = a). Then (vecs w=⟨a−1,a+1,a⟩) would also represent these vectors.
52) Solve the equation (vecs w×⟨1,0,−1⟩=⟨3,0,3⟩,) where (vecs w=⟨w_1,w_2,w_3⟩) is a nonzero vector with a magnitude of ( 3).
53) [T] A mechanic uses a 12-in. wrench to turn a bolt. The wrench makes a ( 30°) angle with the horizontal. If the mechanic applies a vertical force of ( 10) lb on the wrench handle, what is the magnitude of the torque at point ( P) (see the following figure)? Express the answer in foot-pounds rounded to two decimal places.
- Answer:
- 8.66 ft-lb
54) [T] A boy applies the brakes on a bicycle by applying a downward force of 20 lb on the pedal when the 6-in. crank makes a ( 40°) angle with the horizontal (see the following figure). Find the torque at point ( P). Express your answer in foot-pounds rounded to two decimal places.
55) [T] Find the magnitude of the force that needs to be applied to the end of a 20-cm wrench located on the positive direction of the y-axis if the force is applied in the direction ( ⟨0,1,−2⟩) and it produces a ( 100) N·m torque to the bolt located at the origin.
- Answer:
- 250 N
56) [T] What is the magnitude of the force required to be applied to the end of a 1-ft wrench at an angle of ( 35°) to produce a torque of ( 20) N·m?
57) [T] The force vector (vecs F) acting on a proton with an electric charge of ( 1.6×10^{−19},C) (in coulombs) moving in a magnetic field (vecs B) where the velocity vector (vecs v) is given by (vecs F=1.6×10^{−19}(vecs v×vecs B)) (here, (vecs v) is expressed in meters per second, (vecs B) is in tesla [T], and (vecs F) is in newtons [N]). Find the force that acts on a proton that moves in the (xy)-plane at velocity (vecs v=10^5hatimath+10^5hatjmath) (in meters per second) in a magnetic field given by (vecs B=0.3hatjmath).
- Answer:
- (vecs F=4.8×10^{−15},kN)
58) [T] The force vector (vecs F) acting on a proton with an electric charge of ( 1.6×10^{−19},C) moving in a magnetic field (vecs B) where the velocity vector (vecs v) is given by (vecs F=1.6×10^{−19}(vecs v×vecs B)) (here, (vecs v) is expressed in meters per second, (vecs B) in ( T), and (vecs F) in ( N)). If the magnitude of force (vecs F) acting on a proton is ( 5.9×10^{−17},N) and the proton is moving at the speed of 300 m/sec in magnetic field (vecs B) of magnitude 2.4 T, find the angle between velocity vector (vecs v) of the proton and magnetic field (vecs B). Express the answer in degrees rounded to the nearest integer.
59) [T] Consider (vecs r(t)=⟨cos t,sin t,2t⟩) the position vector of a particle at time ( t∈[0,30]), where the components of (vecs r) are expressed in centimeters and time in seconds. Let ( vecd{OP}) be the position vector of the particle after ( 1) sec.
a. Determine unit vector (vecs B(t)) (called the binormal unit vector) that has the direction of cross product vector (vecs v(t)×vecs a(t),) where (vecs v(t)) and (vecs a(t)) are the instantaneous velocity vector and, respectively, the acceleration vector of the particle after ( t) seconds.
b. Use a CAS to visualize vectors (vecs v(1),vecs a(1)), and (vecs B(1)) as vectors starting at point ( P) along with the path of the particle.
a. (vecs B(t)=⟨frac{2sqrt{5}sin t}{5},−frac{2sqrt{5}cos t}{5},frac{sqrt{5}}{5}⟩;)
b.
60) A solar panel is mounted on the roof of a house. The panel may be regarded as positioned at the points of coordinates (in meters) ( A(8,0,0), B(8,18,0), C(0,18,8),) and ( D(0,0,8)) (see the following figure).
a. Find vector (vecs n=vecd{AB}×vecd{AD}) perpendicular to the surface of the solar panels. Express the answer using standard unit vectors. Note that the magnitude of this vector should give us the area of rectangle (ABCD).
b. Assume unit vector (vecs s=frac{1}{sqrt{3}}hatimath+frac{1}{sqrt{3}}hatjmath+frac{1}{sqrt{3}}hat{k}) points toward the Sun at a particular time of the day and the flow of solar energy is (vecs F=900vecs s) (in watts per square meter [( W/m^2)]). Find the predicted amount of electrical power the panel can produce, which is given by the dot product of vectors (vecs F) and (vecs n) (expressed in watts).
c. Determine the angle of elevation of the Sun above the solar panel. Express the answer in degrees rounded to the nearest whole number. (Hint: The angle between vectors (vecs n) and (vecs s) and the angle of elevation are complementary.)
Contributors
Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.
Exercises and LaTeX edited by Paul Seeburger
Section11.4Applications of Double Integrals
So far, we have interpreted the double integral of a function (f) over a domain (D) in two different ways. First, (iint_D f(x,y) , dA) tells us a difference of volumes — the volume the surface defined by (f) bounds above the (xy)-plane on (D) minus the volume the surface bounds below the (xy)-plane on (Dtext{.}) In addition, (frac{1}{A(D)} iint_D f(x,y) , dA) determines the average value of (f) on (Dtext{.}) In this section, we investigate several other applications of double integrals, using the integration process as seen in Preview Activity 11.4.1: we partition into small regions, approximate the desired quantity on each small region, then use the integral to sum these values exactly in the limit.
The following preview activity explores how a double integral can be used to determine the density of a thin plate with a mass density distribution. Recall that in single-variable calculus, we considered a similar problem and computed the mass of a one-dimensional rod with a mass-density distribution. There, as here, the key idea is that if density is constant, mass is the product of density and volume.
Subsection11.4.1Mass
Density is a measure of some quantity per unit area or volume. For example, we can measure the human population density of some region as the number of humans in that region divided by the area of that region. In physics, the mass density of an object is the mass of the object per unit area or volume. As suggested by Preview Activity 11.4.1, the following holds in general.
Subsection11.4.2Area
If we consider the situation where the mass-density distribution is constant, we can also see how a double integral may be used to determine the area of a region. Assuming that (delta(x,y) = 1) over a closed bounded region (Dtext{,}) where the units of (delta) are “mass per unit of area,” it follows that (iint_D 1 , dA) is the mass of the lamina. But since the density is constant, the numerical value of the integral is simply the area.
As the following activity demonstrates, we can also see this fact by considering a three-dimensional solid whose height is always 1.
We now formally state the conclusion from our earlier discussion and Activity 11.4.3.
Subsection11.4.3Center of Mass
The center of mass of an object is a point at which the object will balance perfectly. For example, the center of mass of a circular disk of uniform density is located at its center. For any object, if we throw it through the air, it will spin around its center of mass and behave as if all the mass is located at the center of mass.
In order to understand the role that integrals play in determining the center of a mass of an object with a nonuniform mass distribution, we start by finding the center of mass of a collection of (N) distinct point-masses in the plane.
Let (m_1text{,}) (m_2text{,}) (ldotstext{,}) (m_N) be (N) masses located in the plane. Think of these masses as connected by rigid rods of negligible weight from some central point ((x,y)text{.}) A picture with four masses is shown in Figure 11.4.3. Now imagine balancing this system by placing it on a thin pole at the point ((x,y)) perpendicular to the plane containing the masses. Unless the masses are perfectly balanced, the system will fall off the pole. The point ((overline{x}, overline{y})) at which the system will balance perfectly is called the center of mass of the system. Our goal is to determine the center of mass of a system of discrete masses, then extend this to a continuous lamina.
Each mass exerts a force (called a moment) around the lines (x=overline{x}) and (y=overline{y}) that causes the system to tilt in the direction of the mass. These moments are dependent on the mass and the distance from the given line. Let ((x_1,y_1)) be the location of mass (m_1text{,}) ((x_2,y_2)) the location of mass (m_2text{,}) etc. In order to balance perfectly, the moments in the (x) direction and in the (y) direction must be in equilibrium. We determine these moments and solve the resulting system to find the equilibrium point ((overline{x}, overline{y})) at the center of mass.
The force that mass (m_1) exerts to tilt the system from the line (y=overline{y}) is
where (g) is the gravitational constant. Similarly, the force mass (m_2) exerts to tilt the system from the line (y= overline{y}) is
In general, the force that mass (m_k) exerts to tilt the system from the line (y= overline{y}) is
For the system to balance, we need the forces to sum to 0, so that
Solving for (overline{y}text{,}) we find that
A similar argument shows that
The value (M_x~=~sum_{k=1}^N m_ky_k) is called the total moment with respect to the (x)-axis; (M_y~=~sum_{k=1}^N m_kx_k) is the total moment with respect to the (y)-axis. Hence, the respective quotients of the moments to the total mass, (Mtext{,}) determines the center of mass of a point-mass system:
Now, suppose that rather than a point-mass system, we have a continuous lamina with a variable mass-density (delta(x, y)text{.}) We may estimate its center of mass by partitioning the lamina into (mn) subrectangles of equal area (Delta Atext{,}) and treating the resulting partitioned lamina as a point-mass system. In particular, we select a point ((x_{ij}^*,y_{ij}^*)) in the (ij)th subrectangle, and observe that the quanity
is density times area, so (delta(x_{ij}^*,y_{ij}^*) Delta A) approximates the mass of the small portion of the lamina determined by the subrectangle (R_{ij}text{.})
We now treat (delta(x_{ij}^*,y_{ij}^*) Delta A) as a point mass at the point ((x_{ij}^*,y_{ij}^*)text{.}) The coordinates ((overline{x}, overline{y})) of the center of mass of these (mn) point masses are thus given by
If we take the limit as (m) and (n) go to infinity, we obtain the exact center of mass ((overline{x}, overline{y})) of the continuous lamina.
The center of mass of a lamina can then be thought of as a weighted average of all of the points in the lamina with the weights given by the density at each point. The centroid of a lamina is the just the average of all of the points in the lamina, or the center of mass if the density at each point is 1.
The numerators of (overline{x}) and (overline{y}) are called the respective moments of the lamina about the coordinate axes. Thus, the moment of a lamina (D) with density (delta = delta(x,y)) about the (y)-axis is
11.4 Perpendicular Cross Sectionsap Calculus Solver
and the moment of (D) about the (x)-axis is
If (M) is the mass of the lamina, it follows that the center of mass is
Subsection11.4.4Probability
Calculating probabilities is a very important application of integration in the physical, social, and life sciences. To understand the basics, consider the game of darts in which a player throws a dart at a board and tries to hit a particular target. Let us suppose that a dart board is in the form of a disk (D) with radius 10 inches. If we assume that a player throws a dart at random, and is not aiming at any particular point, then it is equally probable that the dart will strike any single point on the board. For instance, the probability that the dart will strike a particular 1 square inch region is (frac{1}{100 pi}text{,}) or the ratio of the area of the desired target to the total area of (D) (assuming that the dart thrower always hits the board itself at some point). Similarly, the probability that the dart strikes a point in the disk (D_3) of radius 3 inches is given by the area of (D_3) divided by the area of (Dtext{.}) In other words, the probability that the dart strikes the disk (D_3) is
The integrand, (frac{1}{100pi}text{,}) may be thought of as a distribution function, describing how the dart strikes are distributed across the board. In this case the distribution function is constant since we are assuming a uniform distribution, but we can easily envision situations where the distribution function varies. For example, if the player is fairly good and is aiming for the bulls eye (the center of (D)), then the distribution function (f) could be skewed toward the center, say
for some constant positive (Ktext{.}) If we assume that the player is consistent enough so that the dart always strikes the board, then the probability that the dart strikes the board somewhere is 1, and the distribution function (f) will have to satisfy 1
For such a function (ftext{,}) the probability that the dart strikes in the disk (D_1) of radius 1 would be
Indeed, the probability that the dart strikes in any region (R) that lies within (D) is given by
The preceding discussion highlights the general idea behind calculating probabilities. We assume we have a joint probability density function (ftext{,}) a function of two independent variables (x) and (y) defined on a domain (D) that satisfies the conditions
(f(x,y) geq 0) for all (x) and (y) in (Dtext{,})
the probability that (x) is between some values (a) and (b) while (y) is between some values (c) and (d) is given by
begin{equation*}int_a^b int_c^d f(x,y) , dy , dx,end{equation*}The probability that the point ((x,y)) is in (D) is 1, that is
begin{equation}iint_D f(x,y) , dA = 1.label{eq_11_4_pdf}tag{11.4.1}end{equation}
Note that it is possible that (D) could be an infinite region and the limits on the integral in Equation (11.4.1) could be infinite. When we have such a probability density function (f=f(x,y)text{,}) the probability that the point ((x,y)) is in some region (R) contained in the domain (D) (the notation we use here is “(P((x,y)in R))”) is determined by
11.4 Perpendicular Cross Sectionsap Calculus Equation
Subsection11.4.5Summary
11.4 Perpendicular Cross Sectionsap Calculus Formulas
The mass of a lamina (D) with a mass density function (delta = delta(x,y)) is (iint_D delta(x,y) , dA.)
The area of a region (D) in the plane has the same numerical value as the volume of a solid of uniform height 1 and base (Dtext{,}) so the area of (D) is given by (iint_D 1 , dA.)
The center of mass, ((overline{x},overline{y})text{,}) of a continuous lamina with a variable density (delta(x,y)) is given by
begin{equation*}overline{x} = frac{iint_D xdelta(x,y) , dA}{iint_D delta(x,y) , dA} text{ and } overline{y} = frac{iint_D ydelta(x,y) , dA}{iint_D delta(x,y) , dA}.end{equation*}Given a joint probability density function (f) is a function of two independent variables (x) and (y) defined on a domain (Dtext{,}) if (R) is some subregion of (Dtext{,}) then the probability that ((x,y)) is in (R) is given by
begin{equation*}iint_R f(x,y) , dA.end{equation*}
11.4 Perpendicular Cross Sections Ap Calculus Multiple Choice
Exercises11.4.6Exercises
